Weighted average is a calculation that considers the importance of different numbers in a given data set. To calculate the weighted average, each number in the set is multiplied by a predetermined weight.
It’s generally more accurate than simple averaging which only assigns the same weight to each of the numbers in the set.
Weighted Average Formula
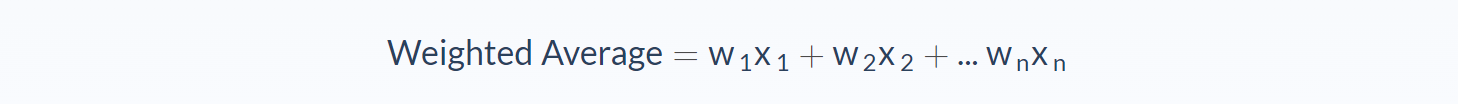
- w = relative weight (%)
- x = value
The arithmetic mean formula is the most commonly used form of simple averaging, where you add each number together and then divide the result by the total amount of numbers in the set. For example 1 + 2 + 3 divided by 3 is an average of 2.
The weighted average formula accounts for the relevancy of each number. So let’s say that 1 only occurs 20% of the time, while 2 and 3 happen 40% of the time. These percentages would be used as the weights and the weighted average would be 2.2
This is a general mathematical formula that is often used in calculating things like test results where answers have different marks available for each question, and it is commonly used in finance as well.
Weighted Average Analysis
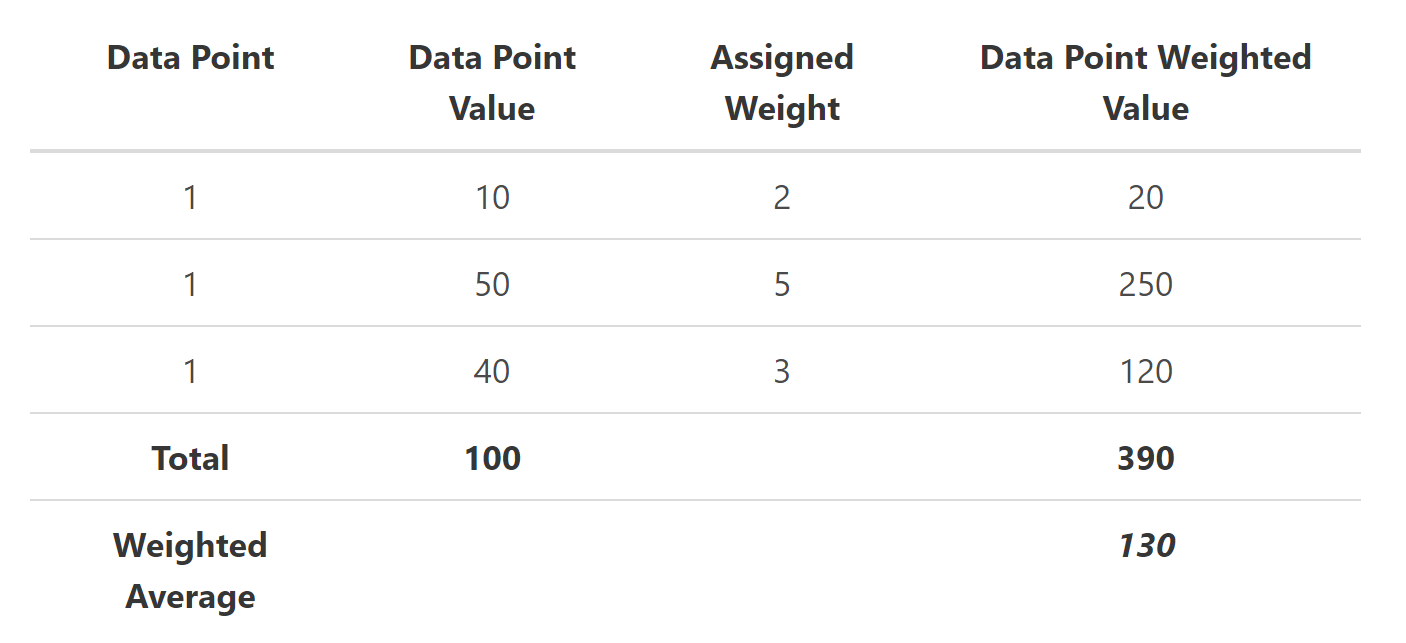
A weighted average is very useful to equalize the frequency of values in a data set. So if you were conducting a study and had enough responses from all age groups to be statistically valid, but one age group had fewer responses relative to their share of the population, you could use a weighted analysis to weight the results so it can be represented proportionally.
The values can also be weighted for different reasons. Let’s say a school want to measure students on attendance, participate and aptitude, you might want to weight the aptitude higher than the others since this is more important to determine how well the student is doing.
Put simply, a weighted average reflects the relative importance of each observed data point and is more descriptive than a simple average, smoothing out the data to enhance the accuracy.
Weighted Average Example
Jonathan is an investor who wants to calculate his rate of return across three different investments. His three investments are:
- Investment A: 25% and 5% return
- Investment B: 25% and 6% return
- Investment C 50% and 8% return
Let’s use the weighted average formula to work out his average return across all investments:
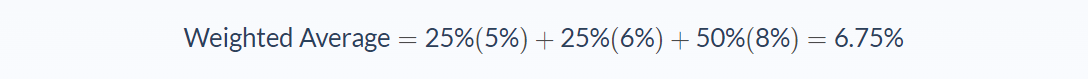
Here we can see that the average return across all investments is 6.75% of whatever the amount is that Jonathan has invested.
Weighted Average Conclusion
When calculating the weighted average, the below points are worth bearing in mind as a quick recap of what it is, why it’s used, and how to use it:
- Weighted Average is a calculation that considers the importance of different numbers in a given data set.
- Weighted Average Formula is used in several finance-related problems.
- A weighted average is regularly used to balance the recurrence of the qualities in an informational index or data set.
- Investors calculate the weighted average of the price that they had paid for their shares.
- Weighted Average is used in portfolio returns, valuation and inventory accounting.
- To discern whether the shares of the companies are correctly priced during an evaluation, investors utilize the weighted average cost of capital (WACC) in order for the company’s cash flows to be discounted.
Weighted Average Calculator
You can use the weighted average calculator below to work out your own weighted average for financial investments by entering the weight and the return rate. Note: if the weight is a percentage, enter it as decimal (i.e. 25% is 0.25).
FAQs
1. What is a weighted average?
A weighted average is a calculation that considers the importance of different numbers in each data set. This is often done by giving each number a weight, which can be based on its occurrence frequency or some other value. The weighted average formula is used in several finance-related problems, including portfolio returns, valuation and inventory accounting.
2. What is the difference between average and weighted average?
While a simple average is just the sum of all the numbers divided by how many there are, a weighted average takes the importance of each number into account. This is done by giving each number a weight, which can be based on its occurrence frequency or some other value. As a result, the weighted average is more descriptive than the simple average and provides a more accurate representation of the data set.
3. Why would you use a weighted average?
There are a few reasons you might want to use a weighted average. One is when you want to balance the recurrence of the qualities in an informational index or data set. Another is when you're trying to discern whether the shares of the companies are correctly priced during an evaluation, and you need to use the weighted average cost of capital (WACC) for the company's cash flows to be discounted. Investors might also use a weighted average when calculating their portfolio returns or valuations.
4. How is a weighted average calculated?
There's no one definitive way to calculate a weighted average, as it can be done in different ways depending on the problem you're trying to solve. However, the most common approach is to use the weighted average formula, which considers the importance of each number by giving it weight. You can then use this weighted average value to get a more accurate representation of the data set.
5. What is an example of a weighted average?
One example of a weighted average is when investors calculate the weighted average of the price that they had paid for their shares. This considers not just the number of shares that they own, but also the price at which they bought them. As a result, it gives a more accurate picture of how their investment has performed.
